How To Find The Concavity Of A Function
![]()
-
Notice the second derivative of f.

-
Set the second derivative equal to nil and solve.
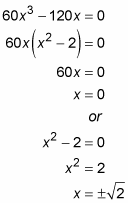
-
Determine whether the 2d derivative is undefined for any ten-values.

Steps ii and 3 requite you lot what you could call "2nd derivative critical numbers" of f because they are analogous to the critical numbers of f that you find using the first derivative. But this set of numbers has no special name. In any effect, the important thing to know is that this listing is fabricated up of the zeros of f ′′ plus any x-values where f ′′ is undefined.
-
Plot these numbers on a number line and examination the regions with the second derivative.
Apply –2, –1, 1, and 2 as examination numbers.
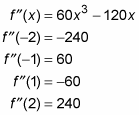
Because –2 is in the left-about region on the number line below, and considering the second derivative at –two equals negative 240, that region gets a negative sign in the figure below, and so on for the other 3 regions.

A second derivative sign graph
A positive sign on this sign graph tells you that the part is concave up in that interval; a negative sign means concave down. The function has an inflection point (usually) at any x-value where the signs switch from positive to negative or vice versa.
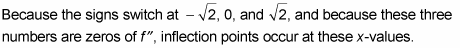
If you go a problem in which the signs switch at a number where the second derivative is undefined, you accept to check one more affair before last that there's an inflection point there. An inflection bespeak exists at a given 10-value simply if there is a tangent line to the role at that number. This is the case wherever the get-go derivative exists or where in that location's a vertical tangent.
-
Plug these iii x-values into f to obtain the function values of the 3 inflection points.
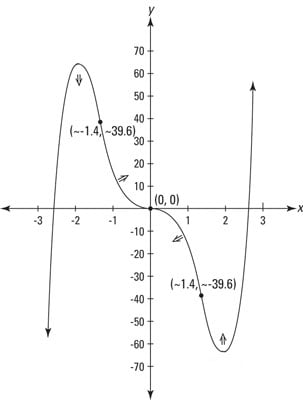
A graph showing inflection points and intervals of concavity
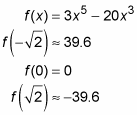
The foursquare root of two equals about 1.4, so in that location are inflection points at about (–1.4, 39.half-dozen), (0, 0), and almost (one.4, –39.6).
Near This Article
This commodity can be found in the category:
- Calculus ,
Source: https://www.dummies.com/article/academics-the-arts/math/calculus/how-to-locate-intervals-of-concavity-and-inflection-points-192163/
Posted by: wigginscolusay.blogspot.com

0 Response to "How To Find The Concavity Of A Function"
Post a Comment